Some differential equations examples
Example 1
One known case is the differential equation second order second degree let say (d2y/(dx1dx2))2+4×1=0 or differential equation second order second degree self dependence (d2y/(dx1dx2))2+sin(y2)+2(y2)3=0, both are solvable numerically, the first is solvable analytically i.e. could have an exact solution but with the second equation only a value as a result of the numerical is the solution. The first designer with first equation can draw profiles and make induction and make appropriate move toward his target where the second designer with the second equation cannot, what if the first designer was with the second equation, he cannot make the appropriate moves too. Second example of difficulty (d2y/(dx1dx2))2/(dy/dx3)+4×1=0, in the first term of the equation the enumerator and the denumerator are differential of the dependent function.
Example 2
As an important example, is the differential equation fifth order third degree, we want here to distinguish between two fundamentals first the differential equation is easy let say we have three independents namely x1,x2,x3, (d5y/dx1dx2(d3x3))3+x1=0, the second with the same independents (d5y/dx1dx2(d3x3))3/(d3y/d3x3)+len(y)-4y3=0 which is not easy, iteration is needed with error handling computed many times numerically to figure out values not even enough to know any profile and we have a nonfigurative attribution case.
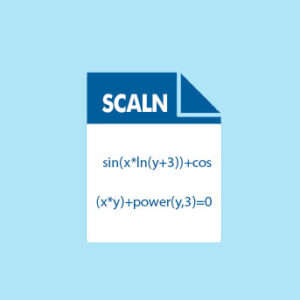
Example 3
A simple example of related, the two cases, first the integration y=Ssin(x)cos(x)dx which is easy having the exact solution y=f(x) of course able to make insights about f(x) and the second y=Ssin(x)cos(y)dx which is not easy; actually you need to walk from and back between iteration and management error and compute many times numerically making the required computer programs too and at the end you have the appropriate table of data using it carefully, these tables, to figure out an insight to make a plan out of a group of plans to make the figured out change of the argument.