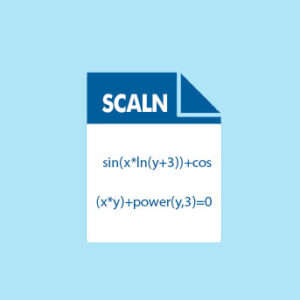
Sometimes, equations include the dependent function on both sides, dazzling in all simplified steps and appearing in many formats. This complexity often leads to significant challenges in iteration and error computation, especially when the equation diverges.
When divergence occurs, the equation moves away from a solution rather than converging towards one.
This divergence makes it nearly impossible to catch a solution using traditional methods.
Handling such equations requires sophisticated numerical techniques and computational tools.
Iterative methods, such as the Newton-Raphson method, can approximate solutions but need careful management of initial guesses and error thresholds to ensure convergence. If these methods fail to converge, significant computational challenges arise, rendering the problem intractable without advanced strategies.
Accurate error estimation is crucial in these scenarios. It helps gauge the reliability of the solution and determine whether the iterations are progressing toward the desired outcome. If the error increases or fluctuates wildly, it signals divergence, prompting a reassessment of the approach or even the formulation of the problem.
In summary, equations with the dependent function on both sides represent a formidable challenge in mathematical problem-solving. Convergence issues and error computations add layers of complexity, often requiring a combination of theoretical insight and practical numerical methods to navigate successfully.
