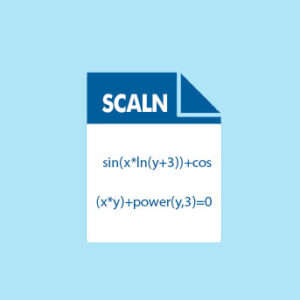
Design and integral equation
The Art of Design and Integral Equations
Design and mathematics might seem worlds apart, but integral equations play a pivotal role in many design processes. From engineering structures to optimizing systems, understanding and solving integral equations can lead to innovative and efficient designs.
Design, the very important verbal phase in manufacturing, to do so you walk from the physics phase then the mathematics phase then go between the both end up with the model, of course you meet such an integration or a differential equation or more of which can easy carry out or not.
What Are Integral Equations?
Integral equations involve functions under an integral sign, where the unknown function appears in the equation itself. They are essential in various fields, including physics, engineering, and economics, as they describe phenomena involving accumulation or distribution over a domain.
Importance in Design
In engineering, integral equations help model systems where the effect at any point depends on the accumulation of contributions from other points. For instance, in structural engineering, they can describe how stress and strain distribute throughout a material.
Solving Integral Equations
Solutions to integral equations often require numerical methods. Techniques such as the trapezoidal rule, Simpson’s rule, and Gaussian quadrature are commonly employed. Software tools like MATLAB and Mathematica provide robust environments for solving these equations.