Curve fitting of function of multiple arguments
Curve Fitting of Functions with Multiple Arguments
Curve fitting is a crucial technique in data analysis, where we model the relationship between dependent and independent variables. When dealing with functions of multiple arguments, this process becomes more complex but equally valuable. Let’s dive into the essentials of curve fitting for functions with multiple arguments.
With mlti needs the minimum number of points and have the nearest solution fit polynomial
Doing curve fitting is crucial in mathematics in the world of numerical forest to proceed forwards in design or at least having solutions
Many softwares requires at least number of points to do this curve fitting but mlti requires at least 1.
Understanding the Basics
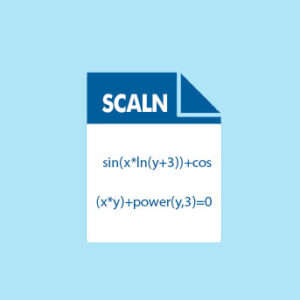
Curve fitting involves finding a mathematical function that best describes the relationship between input variables (independent) and output variables (dependent). When our function has multiple arguments, it takes the form f(x1,x2,…,xn)f(x_1, x_2, …, x_n)f(x1,x2,…,xn).
Single or Multiple Variables Function Iteration
Adaptive iteration techniques ensure that solutions converge more reliably, making the process of finding roots or optimizing functions more efficient.
Conclusion
Curve fitting for functions with multiple arguments is a sophisticated yet essential technique in data analysis. By selecting the right model, preparing your data, implementing fitting techniques, and evaluating the fit, you can uncover valuable insights from your data. Happy fitting!